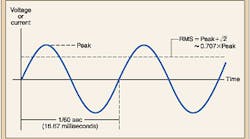
We all know that electric utilities in the United States generate electricity at various 60-Hz voltage levels that result in a sine wave waveform. The height, or amplitude, of this sine wave is called its “peak value.” Instead of using the peak value, however, we describe the amplitude of a sine wave as its effective, or root-mean-square (rms), value.
One key parameter derived from the two values mentioned above is crest factor, which is the ratio of the peak value to the root-mean-square (rms) value of a waveform. This is expressed by the equation C = XPEAK ÷ XRMS. For a pure sine wave (Figure), the peak is 1.0, and the rms value is 0.707. Thus, the crest factor of a pure sine wave is 1.414 (1.0 ÷ 0.707).
It's interesting to note that crest factor is also an important parameter in mechanical systems. According to the paper, “What is ‘Crest Factor’ and Why Use It,” by Alan Friedman of DLI Engineering, Bainbridge Island, Wash., crest factor can indicate rolling element bearing wear, gear tooth wear, or cavitation. Quite often, it's trended over time to see if the amount of impacting is increasing or not.
DC voltages have a crest factor of 1.0, because the rms and peak amplitudes are equal. It's the same for a square wave (50% duty cycle). For a triangle wave, it's 1.73. Crest factors for other waveforms are shown in the Table (click here to see Table). As you can see, crest factor is a dimensionless quantity.
Measurement instrument performance
The IEEE dictionary has a somewhat different definition of crest factor — one that can be attributed to average reading or rms voltmeters: “The ratio of the peak voltage value that an average reading or root-mean-square voltmeter will accept without overloading to the full scale value of the range being used for measurement.”
Crest factor is an important parameter to understand when you're trying to take accurate measurements of low-frequency signals. For example, suppose you have a digital multimeter with an AC accuracy of 0.03%, which is always specified for sine waves, and an additional error of 0.2% for crest factors between 1.414 and 5.0. If you're measuring a triangular wave, which has a crest factor of 1.73, then the total error using this multimeter would be 0.23% (0.03% + 0.2%).
A true-rms measuring instrument typically has a crest factor performance specification, which relates to the amount of peaking this instrument can measure without error. The higher the performance number, the better the performance of the device. You'll find these specification numbers in the range of 2.0 to 7.0.
A typical DMM will have a crest factor number of 3.0 at full scale, which is adequate for most distribution measurements. At half scale, the crest factor is double, or 6.0. For example, some meters will have a crest factor specification of 3.0 when measuring 300VAC and 6.0 when measuring 150VAC.
Wattmeter accuracy is also susceptible. According to Donald E. Lancaster, an author, inventor, and microcomputer pioneer best known for his column, “Tech Musing,” which appeared in Electronics Now and Poptronix magazines, the ratio of rms to average current will change wildly from waveform to waveform and is very much duty cycle dependent, as noted in the June 1998 edition of his column. He goes on to say that every wattmeter design has its specific maximum allowable crest factor. Crest factors above this critical value will usually read low (possibly severely so). Finally, he states that most ordinary wattmeters are totally unsuited to accurately measure lower duty cycle waveforms with high crest factors and recommends that you check the wattmeter's crest factor limit to be sure.
The difference between peak and rms readings
When taking field measurements with a digital multimeter, it's important to understand the difference between peak and rms readings. As previously stated, the term “crest factor” describes the ratio of the peak value of a measured waveform to its rms value. This crest factor value will vary from 1.414 (value for a pure sine wave) if the waveform is distorted. That's because the distortion results in a peak value that's different than that of a pure, undistorted sine wave. Therefore, a “good” sine wave will have a peak value that's close to 1.414 times its true rms value.
This is where the “peak capture” capability of a multimeter comes into play. Basically, you use this capability to compare the measured peak value to the theoretical peak value. For example, let's suppose you're measuring voltage on a 120V circuit. Theoretically, the peak value of this voltage waveform would be 169.7V (120V × 1.414). A measured voltage peak, using the peak capture feature, that's significantly higher than this indicates the presence of harmonics.
Be careful here. Many nonlinear loads will cause these peaks to be reduced or clipped, especially if the source impedance is high. Use the following three-step test to verify voltage clipping due to harmonics:
-
Measure the true rms value of the voltage, and then multiply this value by 1.414 to get the theoretical peak value.
-
Measure the actual peak value using the peak-capture feature.
-
Compare the actual value to the theoretical value. If they're significantly different, the waveform is distorted and contains harmonics.
For voltage harmonics, the typical crest factor is below 1.414, as in “flat-top” waveforms, for example. For current harmonics, the typical crest factor is a great deal above 1.414.
Crest factor, computer loads, and power sources
According to the white paper, “Understanding Power Factor, Crest Factor, and Surge Factor,” by American Power Conversion (APC), W. Kingston, R.I., most common electrical appliances exhibit a crest factor of 1.4, as do computers and IT equipment with power factor-corrected power supplies. Other computer loads are unusual in that they exhibit a very high crest factor. For example, personal computers (PCs) and stackable hubs exhibit a crest factor of 2 to 3.
When a load exhibits a crest factor of more than 1.4, the power source, which may be an online UPS, must supply the peak current desired by the load. Any excess peak current will deform the source voltage if the source cannot supply the correct current. Therefore, if you don't size a UPS to supply the crest factor desired by the load, the output voltage waveform of the UPS will be distorted.
The paper goes on to say that most people believe the crest factor of a computer or other electronic load is an inherent characteristic, when in fact, it results from an interaction between the load itself and its AC power source. Basically, the crest factor required by a computer load depends on the AC source's waveform. For a sine wave source, a non-power factor-corrected PC will typically exhibit a crest factor of 2 to 3. For a source waveform that is a stepped approximation to a sine wave — as used in most uninterruptible power supplies rated below 1kW — a PC will exhibit a crest factor of 1.4 to 1.9.
The paper also notes that most people mistakenly believe that it's desirable to operate a computer at as high a crest factor as possible. In fact, computer manufacturers go to great lengths to reduce the crest factor of the computer because high crest factor causes overheating of power supply components.
The reduction in crest factor that occurs when a computer load is operated from a UPS, surge suppressor, or power conditioner is a positive side benefit, unless the reduction is accompanied by excessive distortion of the input voltage waveform to the computer load. Such distortion could possibly result in a significantly reduced peak voltage being supplied to the load, equivalent to a brownout condition.
Typical sine wave UPS systems have crest factor capabilities of approximately 3 when operated at full load, 4 when operated at half load, and 8 when operated at quarter load. Typical smaller stepped-wave models have crest factor capabilities of 1.6 at full load and 2 at half load. UPS systems with this performance will maintain the proper peak voltage into the computer load for computers with any input crest factor specification.
The fact that virtually all equipment installed in data centers today, such as servers, routers, and storage devices, are power factor-corrected has all but eliminated crest factor as a problem in data centers. Instead, crest factor problems today are limited to call center or trading floor installations with a high density of PCs.