The power quality industry has developed certain index values that help us assess the quality of service as it relates to distortion caused by the presence of harmonics. These values, or harmonic indices, serve as a useful metric of system performance. The two most frequently used are total harmonic distortion (THD) and total demand distortion (TDD). They are measures of the effective value of a waveform and can be applied to both current and voltage.
THD
According to the book “Electrical Power Systems Quality” by Dugan, McGranaghan, Santoso, and Beaty (ISBN 0-07-138622-X), THD is a measure of the effective value of the harmonic components of a distorted waveform. It can be calculated for either current or voltage. Although many of today's test and measurement instruments can provide THD values, it's still important to understand the calculation that derives THD. The basic equation is as follows:
where Mh is the rms value of harmonic component h of the quantity M.
Don't be intimidated by this equation. Basically, it's stating that THD is equal to the square root of the sum of the squares of each rms component M from the harmonic after the fundamental (h greater than 1) to the highest harmonic component (hmax), divided by the rms value of the fundamental component (h = 1).
The power quality industry most often uses the THD index to describe voltage harmonic distortion and always references harmonic voltages to the fundamental value of the waveform at the time of measurement, as defined by the equation below:
Basically, this equation is saying voltage THD is equal to the square root of the sum of the squares of each rms harmonic voltage component from the harmonic after the fundamental (h = 2) to the highest harmonic voltage component (h=∞), divided by the rms value of the fundamental voltage component (V1).
This makes sense because the fundamental voltage varies only by a few percent, so any reference of voltage THD, relative to the fundamental, is nearly always a meaningful number.
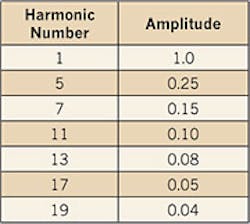
The book “Power Quality in Electrical Systems” by Kusko and Thompson (ISBN-10:0-07-147075-1) provides an example of how to manually calculate THD. Suppose we have a harmonic voltage spectrum as listed in Table 1. Using Equation 1, we can calculate the THD for this scenario:
TDD
As previously mentioned, we can characterize current distortion levels with a THD value, but this can be misleading. According to the book “Electrical Power Systems Quality,” a small current can have a high THD but not be a significant threat. For example, many adjustable-speed drives will exhibit high THD values for the input current while operating at very light loads. This shouldn't be a concern, because the magnitude of harmonic current would be low in this instance, even though its relative current distortion is quite high.
Responding to such scenarios, some analysts have referred to THD as the fundamental of the peak demand load current rather than the fundamental of the present sample. This is called total demand distortion, or TDD. Contrary to popular belief, TDD and not THD serves as the basis for the guidelines in IEEE 519, “Recommended Practices and Requirements for Harmonic Control in Electrical Power Systems.” In fact, IEEE 519 defines TDD as “the total root-sum-square harmonic current distortion, in percent of the maximum demand load current.” The following equation defines TDD:
where IL is the peak or maximum demand load current at the fundamental frequency component measured at the point of common coupling (PCC), which is usually at the customer's metering point. Again, you should not be intimidated by this equation, as it simply states that TDD is equal to the square root of the sum of squares of each of the maximum demand currents from the second harmonic to the maximum harmonic present, divided by the maximum demand load current at the fundamental. TDD is meaningful when monitored at the PCC over a period of time that reflects maximum customer demand. Per IEEE 519, this is typically 15 minutes to 30 minutes.
According to the book “Harmonics and Power Sources” by De La Rosa (ISBN 9780849330162), weak power sources with large demand current, relative to the rated current, will tend to show greater waveform distortion. On the other hand, stiff power sources operating at low demand currents will show decreased waveform distortion.
There are two ways in which to measure IL. With the load already in the system, you can calculate IL as the average of the maximum demand current for the preceding 12 months. Here, you average the 12-month peak demand readings. Or, for a new facility, you can estimate IL by using predicted load profiles.
IEEE 519 and TDD
The main function of IEEE 519 is to control the design of power harmonic filters in electrical systems. It does so by including harmonic voltage limits (Table 10.1, as replicated in Table 2) and current distortion limits (Table 10.3, as replicated in (click here to see Table 3).
Table 10.1 sets the maximum individual frequency voltage harmonics (percent) for loads connected to the PCC, as a function of the size of the load. The measure of size is determined by a ratio called the “short-circuit ratio,” or SCR, which is the ratio of the maximum short-circuit current at the PC (ISC) to the maximum load demand current (IL) of the fundamental at the PCC. As you can see, the reference to the maximum demand load current ties in directly with the definition of TDD. As shown, Table 10.1 sets a harmonic voltage limit of 2.5% to 3% for an SCR of 10 (very large load), down to 0.05% to 0.1% for an SCR of 1,000 (many small loads).
Now, to achieve these voltage harmonic limits, IEEE 519 sets limits (per its Table 10.3) on the amount of harmonic currents injected into the PCC, as a function of the size of the load. For example, for a load with an ISC / IL ratio of 120, the current harmonics less than the 11th must be less than 12% of IL. Also, the TDD must be less than 15%.
Although the concept isn't new, harmonic distortion continues to be a main concern of engineers in the various stages of energy use within the electrical industry. The increasing use of nonlinear loads is keeping harmonic distortion in facility and utility distribution networks on the rise.